
In a triangle ABC, P and Q are the mid points of AB and BC respectively. Point G and H l ie’s on AC such that they are the mid-point of PR and QR respectively. Here R is a point outside the triangle. Then what is the Ratio of area ∆ ABC : area ∆ PQR?
(a) 2 : 3
(b) 5 : 2
(c) 3 : 5
(d) 2 : 1
Sol:
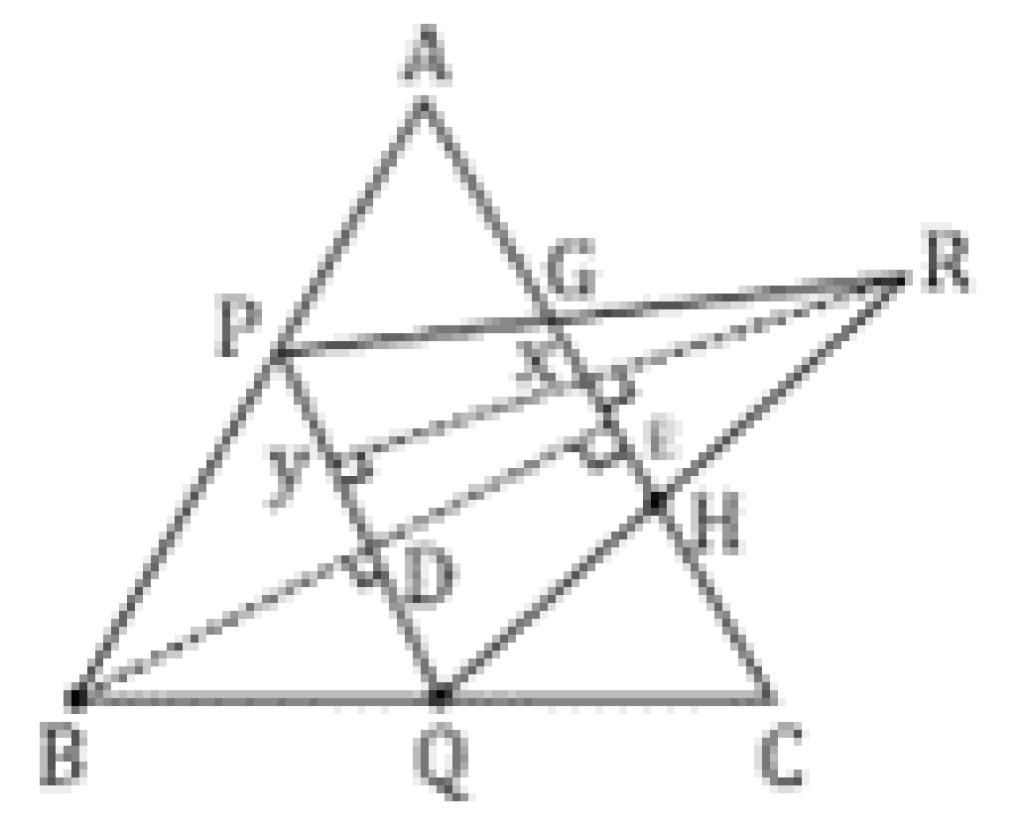
∆ BPQ ~ ∆ BAC
AC = 2PQ
AC II PQ
BE = 2BD
BD = DE
∆ RGH ~∆ RPQ
PQ = 2GH
PQ II GH
RY = 2RX
⇒ RX = XY
Since
RX = XY = BD = DE
RY = BE = h

=2 : 1





