In chemistry solution is referred to as a homogenous mixture of two or more substances in relative amounts that can be varied continuously up to what is called the limit of solubility. The term solution is commonly applied to the liquid state of matter, but solutions of gases and solids are possible.
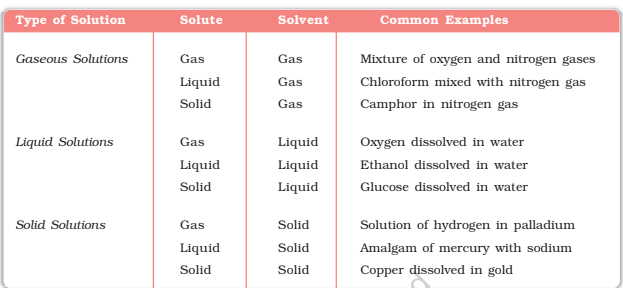
A solution can be categorized into several components. On the basis of physical states of solvent and solute can be categorized as solid, liquid and gaseous solutions. In solid solutions, solute and solvent are in the solid-state.
Composition of a solution can be described by expressing its concentration. The latter can be expressed either qualitatively or quantitatively.
There are several ways by which we can describe the concentration of the solution quantitatively.
(i) Mass percentage (w/w): The mass percentage of a component of a solution is defined as:
Mass % of a component = (Mass of the component in the solution/Total mass of the solution) × 100
(ii) Volume percentage (V/V): The volume percentage is defined as:
Volume % of a component = (Volume of the component/ Total volume of solution) × 100
(ii) Mass by volume percentage (w/V): Another unit which is commonly used in medicine and pharmacy is mass by volume percentage. It is the mass of solute dissolved in 100 mL of the solution.
(iv) Parts per million: When a solute is present in trace quantities, it is convenient to express concentration in parts per million (ppm) and is defined as:
Parts per million = (Number of parts of the component/ Total number of parts of all components of the solution) ×
(v) Mole fraction: Commonly used symbol for mole fraction is x and subscript used on the right hand side of x denotes the component. It is defined as:
Mole fraction of a component = Number of moles of the component/Total number of moles of all the components
Solubility is the ability of a solid, liquid, or gaseous chemical substance (referred to as the solute) to dissolve in solvent (usually a liquid) and form a solution. The solubility of a substance fundamentally depends on the solvent used, as well as temperature and pressure.
Based on the concentration of solute dissolves in a solvent, solutes are categorized into highly soluble, sparingly soluble or insoluble.
Solubility is measured either in grams per 100 g of solvent – g/100 g – or number of moles per 1 L of the solution. Divide the mass of the compound by the mass of the solvent and then multiply by 100 g to calculate the solubility in g/100g.
Solubility is the maximum amount of a substance that will dissolve in a given amount of solvent at a specific temperature.
There are two direct factors that affect solubility: temperature and pressure. Temperature affects the solubility of both solids and gases, but pressure only affects the solubility of gases.
The gas solubility in liquids is greatly affected by temperature and pressure as well as the nature of the solute and the solvent.
There are many gases that readily dissolve in water, while there are gases that do not dissolve in water under normal conditions.
Vapour Pressure of liquid solutions is defined as the pressure exerted by the vapours on the liquid solvent when kept in equilibrium and a certain temperature.
It varies with the nature of liquid and temperature of the surroundings. The pure liquid has more vapour pressure as compared to liquid's solution.
When a binary solution of two volatile liquids is placed in a closed vessel, both the components would evaporate and eventually an equilibrium would be established between vapour phase and the liquid phase.
At a given temperature liquids vaporize. At equilibrium the pressure exertedby the vapour of the liquid over the liquidphase is referred to as vapour pressure. Therefore, Raoult's law turns into a unique instance of Henry's law in which KH get to be equivalent to p10.
Vapour Pressure of liquid solutions is defined as the pressure exerted by the vapours on the liquid solvent when kept in equilibrium and a certain temperature.
It varies with the nature of liquid and temperature of the surroundings.
The pure liquid has more vapour pressure as compared to liquid's solution.
Ideal solution: An ideal solution is a mixture in which the molecules of different species are distinguishable, however, unlike the ideal gas, the molecules in ideal solution exert forces on one another. When those forces are the same for all molecules independent of species then a solution is said to be ideal.
Non-ideal Solution: A non-ideal solution is a solution that does not abide to the rules of an ideal solution where the interactions between the molecules are identical (or very close) to the interactions between molecules of different components.
When we a non-volatile solute in a volatile solvent we observe that there is a decrease in vapour pressure of the solution. These properties depend more on solute particles of the solution and are called colligative properties.
Vapour pressure is the pressure exerted by the vapours over the liquid under the equilibrium conditions at a given temperature.
According to Raoult’s Law:
p1=x1p1o…………………………..(1)
The decrease in vapour pressure of the solvent (∆p1) is given by:
=> ∆p1=p1o-p1
=> ∆p1=p1o-p1ox1 [using equation (1)]
=> ∆p1=p1o (1-x1)
Since we have assumed the solution to be binary solution, x2=1-x1
=> ∆p1=p1ox2
=> x2= ∆p1/p1o
The above equation gives the relative lowering in vapour pressure which is equal to the mole fraction of the solute.
Boiling point elevation refers to the increase in the boiling point of a solvent upon the addition of a solute. When a non-volatile solute is added to a solvent, the resulting solution has a higher boiling point than that of the pure solvent. For example, the boiling point of a solution of sodium chloride (salt) and water is greater than that of pure water.
The boiling point of a solution containing a non-volatile solute can be expressed as follows:
Boiling point of solution = boiling point of pure solvent + boiling point elevation (ΔTb)
The elevation in boiling point (ΔTb) is proportional to the concentration of the solute in the solution. It can be calculated via the following equation.
ΔTb = i*Kb*m
Where,
i is the Van’t Hoff factor
Kb is the ebullioscopic constant
m is the molality of the solute
Freezing point depression refers to the lowering of the freezing point of solvents upon the addition of solutes.
It is a colligative property of solutions that is generally proportional to the molality of the added solute. The depression in the freezing point of a solution can be described by the following formula.
ΔTf = i*Kf*m
Where
ΔTf is the freezing point depression,
i is the Van’t Hoff factor,
Kf is the cryoscopic constant, and
m is the molality.
Osmosis is a process by which the molecules of a solvent pass from a solution of low concentration to a solution of high concentration through a semi-permeable membrane.
There are three different types of solutions: Isotonic Solution, Hypertonic Solution, Hypotonic Solution.
Osmosis is of two types:
Osmotic pressure is the pressure required to stop water from diffusing through a membrane by osmosis. It is determined by the concentration of the solute. Water diffuses into the area of higher concentration from the area of lower concentration. When the concentration of the substances in the two areas in contact is different, the substances will diffuse until the concentration is uniform throughout.
Osmotic pressure can be calculated using the equation:
Π=MRT
where Π denotes the osmotic pressure,
M is the molar concentration of the solute,
R is the gas constant,
T is the temperature
Osmosis influences the transport of nutrients and the release of metabolic waste products.
It is responsible for the absorption of water from the soil and conducting it to the upper parts of the plant through the xylem.
It stabilizes the internal environment of a living organism by maintaining the balance between water and intercellular fluid levels.
It maintains the turgidity of cells.
It is a process by which plants maintain their water content despite the constant water loss due to transpiration.
This process controls the cell to cell diffusion of water.
Osmosis induces cell turgor which regulates the movement of plants and plant parts.
Osmosis also controls the dehiscence of fruits and sporangia.
Higher osmotic pressure protects the plants against drought injury.
Reverse osmosis (RO) is a water purification process that uses a partially permeable membrane to separate ions, unwanted molecules and larger particles from drinking water.
When the water passes through the membrane during the process, the product on the other end is totally clean water without any impurities. Reverse osmosis water is 100% safe to drink.
In chemistry, when the molar masses are calculated and if they are higher or lower than the expected value are known as abnormal molar masses.
The name in itself has abnormal in it which suggests the abnormality of how the molar masses are being calculated, using the Van't Hoff factor.
1. A solution is a homogeneous mixture of two or 9.
more chemically non-reacting substances.
The components of a solution generally cannot be separated by filtration, settling or centrifuging.
2. A solution may be classified as solid, liquid or a gaseous solution.
3. Solubility is defined as the amount of solute in a saturated solution per 100g of a solvent.
4. The solubility of a gas in a liquid depends upon
(a) the nature of the gas and the nature of the liquid,
(b) the temperature of the system, and
(c) the pressure of the gas.
5. The effect of pressure on the solubility of a gas in a liquid is governed by Henry’s Law. It states that the solubility of a gas in a liquid at a given temperature in directly proportional to the partial pressure of the gas Mathematically, P = KHX where P is the partial pressure of the gas; and X is the mole fraction of the gas in the solution and KH is Henry’s Law constant.
6. The vapour pressure of a liquid is the pressure exerted by its vapour when it is in dynamic equilibrium with its liquid, in a closed container.
7. According to Raoults Law, the vapour pressure of a solution containing a non-volatile solute is directly proportional to the mole fraction of the solvent ( XA). The proportionality constant being the vapour pressure of the pure solvent, i.e., P× XA or P = P° XA.
8. A solution which obeys Raoult’s Law at all concentrations and temperatures is known as an ideal solution.
9. Characteristics of an ideal solution:
(a) ∆sol V = 0, i.e., there is no change in volume when an ideal solution is formed.
(b) ∆sol H= 0; i.e., heat is neither evolved nor absorbed during the formation of an ideal solution.
10. (a) The solution shows positive deviation from Raoult’s Law if its vapour pressure is higher than that predicted by Raoult’s Law.
(b) The solution shows negative deviation if its vapour pressure is lower than that predicted by Raoult’s Law.
11. Colligative properties of solutions are those properties which depend only upon the number of solute particles in the solution and not on their nature. Such properties are
(a) Relative lowering in vapour pressure,
(b) Elevation of boiling point,
(c) Depression of freezing point and
(d) Osmotic pressure.
12.

Thus, according to Raoult’s Law, the relative lowering of vapour pressure of a solution is equal to the mole fraction of the solute.
13. For a dilute solution, the elevation in boiling point is found to be proportional to the molality of the
![]()
where ∆Tb is the elevation in boiling point, ‘m’ is the molality and Kb is the Molal elevation constant
14. The depression in freezing point (∆Tf) is proportional to the molality of the solution.
![]()
where Kf is molal depression constant (freezing point depression constant).
15. The spontaneous flow of solvent molecules from a dilute solution into a concentrated solution when the two are separated by a perfect sernipermeable membrane is called osmosis.
16. Osmotic pressure (π) is the pressure which must be applied to the solution side (more concentrated solution) to just prevent the passage of pure solvent into it through a sernipermeable membrane.
Mathematically, π = CRT= nB/V- RT
where n is the osmotic pressure of the solution,
C is the concentration of solution
nB is the number of moles of solute,
V is the volume of the solution in litres,
R is the gas constant, and T is the temperature on the Kelvin scale.
17. Isotonic solutions are those solutions which have the same osmotic pressure. Also they have same molar concentration.
For isotonic solutions, π1 = π2 Also, C1 = C2
18. Van’t Hoff factor, ‘ i’ is used to express the extent of association or dissociation of solutes in solution. It is die ratio of the normal and observed molar masses of the solute, i. e.,

19. In case of association, observed molar mass being more than the normal, the factor ‘T has a value less than one. But in case of dissociation, the van’t Hoff factor is more than one because the observed molar mass has a less value.
20. In case of solutes which do not undergo any association or dissociation in a solvent, the Vant Hoff factor, ‘i’, will be equal to one because the observed and normal molar masses will be same.
21. Inclusion of van’t Hoff factor, ‘F, modifies the equations for colligative properties as follows:
