Electrostatics is referred as the study of electromagnetic phenomena that occur when there are no moving charges.
The electrostatic potential is also known as the electric field potential, electric potential, or potential drop is defined as. The amount of work that is done in order to move a unit charge from a reference point to a specific point inside the field without producing an acceleration.
The electric potential at a point in an electric field is defined as the amount of work done in moving a unit positive charge from infinity to that point along any path when the electrostatic forces are applied. Suppose that a positive charge is placed at a point. The charge placed at that point will exert a force due to the presence of an electric field.
The electric potential at any point at a distance r from the positive charge +q is shown as:
It is given by,
V=14πϵ0qr
Where r is the position vector of the positive charge and q is the source charge.
An electric dipole is an arrangement of two equal and opposite charges separated by a distance 2a. The dipole moment is represented by p which is a vector quantity.
The potential due to a dipole depends on r (distance between the point where potential is calculated and the mid-point of the dipole) and angle between position vector r and dipole moment p.
Dipole potential is inversely proportional to square of r.
For a number of charges present in space, the total potential at a point due to all those charges will be equal to the sum of individual potential of each charge at that point.
Consider a system of charges q1, q2,..., qn with position vectors r1, r2,...,rn relative to some origin. The potential V1 at P due to the charge q1 is
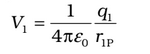
Where r 1P is the distance between q1 and P.
An equipotential surface is a surface with a constant value of potential at all points on the surface. For a single charge q, the potential is given by Eq.
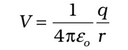
This shows that V is a constant if r is constant. Thus, equipotential surfaces of a single point charge are concentric spherical surfaces centred at the charge.
The relation between electric field and potential are
(i) Electric field is in the direction in which the potential decreases steepest.
(ii) Its magnitude is given by the change in the magnitude of potential per unit displacement normal to the equipotential surface at the point.
The potential energy is characteristic of the current state of configuration and not the way how this configuration was achieved.
The potential for a system of charges is calculated as below:
consider a charge of magnitude q placed in an external electric field of magnitude E. Here the charge q under consideration is very small. The potential energy of the charge q in the field is equal to the work done in bringing the charge from infinity to the point. Here we note that the external electric field E and the corresponding potential energy of the system vary from point to point in the field. Now, we know that the potential at infinity is always taken to be zero; the work done in bringing a charge from infinity to the point is given as qV. The potential energy of the point q at a distance r from the origin in an external electric field is given as, qV(r), where V(r) is the external potential at that point.
When the potential energy of charge(s) is calculated in an external field, following things are understood:
Dipole in an external field experiences a torque which tries to align it in the direction of the electric field. The work done to oppose this force gets stored in the form of potential energy.
The electric field inside a conductor is always zero. So due to which electric potential difference is too zero. In this case total electric potential is zero as net charge inside the conductor is zero. The electrostatic field at different points in a conductor is given below.
Inside Conductor: The electrostatic field inside the conductor is zero. Under no external electric field or static condition, the charge carriers are distributed evenly and there is no electric field inside.
At the surface of a charged conductor: The electrostatic field at the surface of a charged conductor is normal to the surface at every point. For a non-normal Electric field, there is a non-zero component along the normal. Therefore, Electric field should have no tangential component in static.
Interior of a conductor: There is no electrostatic field in the interior of the conductor. All the excess charge resides at the surface. Under static conditions, the excess charge resides at the surface of the conductor. On a closed surface, the electrostatic field is zero. So from gauss’s law, there is no net charge enclosed by the surface.
Throughout the Volume of the conductor: The Electrostatic potential is constant throughout the volume of the conductor and is equal to its value on surface. Since, conductor has no tangential component; no work is done in moving charge within conductor and on its surface. Hence the potential is constant.
Dielectrics are non-conducting substances which are the insulating materials and are bad conductor of electric current. Dielectric materials can be made to hold an electrostatic charge while dissipating minimal energy in the form of heat. Examples of dielectric are Mica, Plastics, Glass, Porcelain and Various Metal Oxides and even dry air is also example of dielectric.
A dielectric develops a net dipole moment in the presence of an external field.The dipole moment per unit volume is called polarization and is denoted by P. For linear isotropic dielectrics (substances where induced dipole moment is in the direction of the field and is proportional to the field strength), P = χe E
Here, Χe means electric susceptibility of the dielectric medium.
A capacitor is a system of two conductors separated by an insulator. The conductors have charges, say Q1 and Q2, and potentials V1 and V2. Usually, in practice, the two conductors have charges Q and – Q, with potential difference V = V1– V2 between them.
A capacitor is a system of two conductors separated by an insulator. The total charge of a capacitor is zero while the conductors have charge Q and –Q. A single conductor can be considered as capacitor with other conductor at infinity. Electric field in the region between the conductors is proportional to the charge Q.
Capacitanceis denoted by, C = Q/V. It depends on:
SI unit of capacitance is F (Farad).
A parallel plate capacitor consists of two large plane parallel conducting plates separated by a small distance. Electric field inside the capacitor has a direction from positive to negative plate. For very small‘d’, the electric field is considered as uniform. For large‘d’, the electric field is non-uniform and it bends around the corners of the plate which is called fringing of the field.
When a dielectric is present between the plates of a parallel plate capacitor fully occupying the region, the dielectric is polarized by the electric field. The surface charge densities are considered as σp and -σp. Dielectric constant of a substance is the factor by which the capacitance increases from its vacuum value, when the dielectric is fully inserted in between the plates of the capacitor
We can combine several capacitors of capacitance C1, C2,..., Cn to obtain a system with some effective capacitance C. The effective capacitance depends on the way the individual capacitors are combined. Two simple possibilities are discussed below.
Capacitors are said to be connected in series when the second plate of a capacitor is connected to the first plate of the next capacitor and so on. Capacitors are connected in series as per the below diagram. The charge across the arrangement will remain the same. The total potential drop is the sum of individual potential drops across each capacitor. The inverse of total capacitance is the sum of inverse of individual capacitances.
Capacitors are said to be connected in parallel when the first and second plate of a capacitor is connected to the first and second plate of the next capacitor respectively. Capacitors are connected in parallel as per the below diagram. The potential across the arrangement will remain the same. The total charge is the sum of individual charges across each capacitor. The total capacitance is sum of individual capacitances.
Energy is stored in the capacitor when work is done to move a positive charge from negative conductor towards the positive conductor against the repulsive force.
The energy U stored in a capacitor of capacitance C, with charge Q and voltage V is
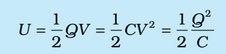
The electric energy density (energy per unit volume) in a region with electric field is (1/2)ε0E2.
| Article and Schedule Quiz | Start Test! |