Discuss the optimum harvesting of Renewable resource.
⇒The renewable resource has the capacity to regenerating itself. Here we will discuss about the optimum level of harvesting of a renewable resource. For simplicity, we are talking the example of fish.
We know that the renewable resources follows a logistic growth function. The growth of resources stock fish is a function of the size of the stock itself, in the absence of any change in the ecological characteristic of the ecosystem. The biological growth ‘G’ of the fish may be functionally expressed as G=G (S). Where ‘S’ the stock size. In the following diagram the logistic growth function has
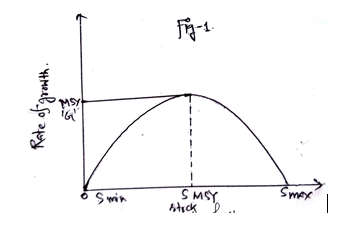
Here,
Smin is the minimum stock of the resource
Smax is the maximum stock of the resource
SMSY : Maximum sustainable yield stock level of fish.
MSY: the largest perpetual yield that could be obtain from the resource.
On the vertical axis, we have measured rate of growth of the fish (annual) and on the horizontal axis, fish stock is measured. Here, if the rate of harvest is equal to MSY, the resources will forever and we can get maximum from it each period. But, MSY doesn’t correspond to the optimum harvest rates. We need to introduce rate of harvesting and the cost and revenge related to harvesting the resource, to determine the optimum yield.
So, to do this, we have to introduce a relation between effort, harvest and stock level. The relationship is E=H/S, where ‘S’ is the stock, it is the actual harvest and ‘E’ is effort level.
For example, if in a pond of 300, 100 are caught the effort level is E=100/200 = 1/2. So, bigger the effort greater proportion of stock that would be harvested. In the following diagram (2) we have shown different effort levels=(E1 & E2). An effort level of e different effort levels = (E1 & E2). An effort level of E1 corresponds to a harvest H* and stock S*
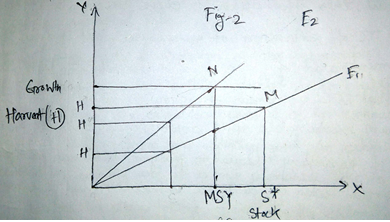
At point ‘M’, the effort level E1 cuts the growth curve effort is equal to the rate of growth of the resource, but H* is not the MSY. To get the MSY, the effort should be increase to E2. This effort level achieves the maximum sustainable yield or harvest at point ‘N’. But again, we have not rightly introduced the concept of cost and revenue and to do this we have to show a relationship between effort and harvest. In the following diagram, the effort-harvest curve in shown—
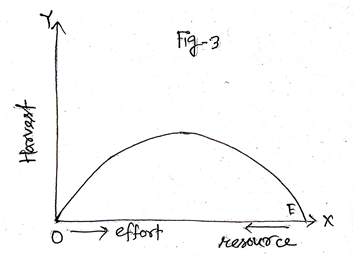
This fig 3 is actually the TR curve, because we know the TR=PX harvest and if we assume the price of the resource remains constant, this effort harvest curve become the TR curve.
Now, the TC of extraction of a resource will induce the wages, equipment and cost and reward for risk. Let us assume that TC is linear function.
The optimum sustainable yield corresponds to that level where marginal cost of harvesting is equal o marginal revenue from harvesting. We also know that when the distance between TR and TC maximum, then also optimum level is attained. In the following diagram, the maximum distance between TR and TC occurs at point ‘E’ and therefore OE is the optimum sustainable yield.
The above explanation is a static model, but in actual practice, there always exists change factor. Therefore, we have to make it dynamic and to do this, the future cost and benefits are discounted. The basic rule for exploration of a renewable resource when future benefits and cost are discounted is---
Biological growth rates (b)+ growth capital value (c) = discounted rate (d). If the discount rate is greater than btc (d>b+c) resource is harvested now. If d < b+c, waiting will be worthwhile and the resource will be harvested later.
| Article and Schedule Quiz | Start Test! |